Example - A current ring
Find the magnetic field on the z-axis produced by a uniform circular current loop of radius R, which carries current I, and which lies in the x-y plane and is centered at the origin.
We shall integrate angle ![]() over
over ![]() in
order to find the total B field. We take the
current to be flowing counterclockwise around the
loop. The magnetic field is along the z-axis, by
symmetry. We take a position z on the
z-axis and a small element of current
in
order to find the total B field. We take the
current to be flowing counterclockwise around the
loop. The magnetic field is along the z-axis, by
symmetry. We take a position z on the
z-axis and a small element of current
![]() on the current loop.
We take the angle between the vector from the
loop to point z and the z-axis to be
on the current loop.
We take the angle between the vector from the
loop to point z and the z-axis to be ![]() .
The cross product
.
The cross product
![]() then has magnitude
then has magnitude ![]() that is
that is
![]() and
and
![]() are perpendicular. The component of Balong the z-axis is
are perpendicular. The component of Balong the z-axis is
![]() putting this
together, we find the magnetic field in the
z-direction to be,
putting this
together, we find the magnetic field in the
z-direction to be,
| (1) |
| (2) |
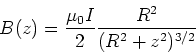 |
(3) |
Time varying electric and magnetic fields
The equations of electrostatics and
magnetostatics are often summarized
as Gauss' law for electrostatics,
| (4) |
| (5) |
| (6) |
To treat time varying fields, we are going to add another term to Ampere's law and we are going to introduce a new law called Faraday's law. The term which is added to Ampere's law was found by Maxwell in 1865. Faraday developed his law in 1831.
- Maxwell's term describes the fact that a time varying electric field induces a magnetic field.
- Faraday's law states that a time varying magnetic field induces an electric field.
These laws together describe the
phenomena of electromagnetic induction.
Faraday's law is more famous
due to it many practical applications
in motors, generators and other
devices. The laws describing these
time varying fields will also
enable us to understand the propagation
of light and other electromagnetic
radiation. In that case we need
both Faraday's law and Maxwell's term.
Maxwell's displacement current
Maxwell noticed that when a
capacitor is charging, there is a logical
inconsistency in Ampere's law. To
understand this inconsistency, consider
an initially uncharged capacitor
connected to a voltage source at t=0.
For simplicity, we consider a parallel
plate capacitor.
Current begins to flow in the circuit
at t=0, charging up the capacitor.
Now we can construct a loop around
the wire in the circuit. However
this loop does not really enclose
the current in the wire. The loop
can pass through the capacitor
without cutting the wire. Therefor
when the capacitor is charging,
Amperes law would state
that there is no enclosed current and
hence the magnetic field is zero.
This is wrong. There is
a magnetic field produced by the
current. Maxwell resolved this
difficulty by adding a new term
which includes the effect of the
electric field which builds up between
the capacitor plates. His idea
was to related this electric field
to the current flowing the circuit.
From Gauss' law, we have,
| (7) |
| (8) |
| (9) |
Example 30-7
A parallel plate capacitor is being charged at i = 1C/s. If the plates are circular with radius, R=0.1m, and are separated by d=1cm, find the magnetic field as a function of distance from the central axis of the capacitor.
Consider a circular loop or radius r centered
on the axis of the capacitor and lying
parallel to the plates. From Ampere's law we have,
| (10) |
| (11) |
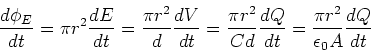 |
(12) |
 |
(13) |
| (14) |