ISP 205, Section 1, Spring 1997
Homework #10
Due: Thursday, April 17, 1997

                                        Name: ________________________


                                        Student Number: ______________
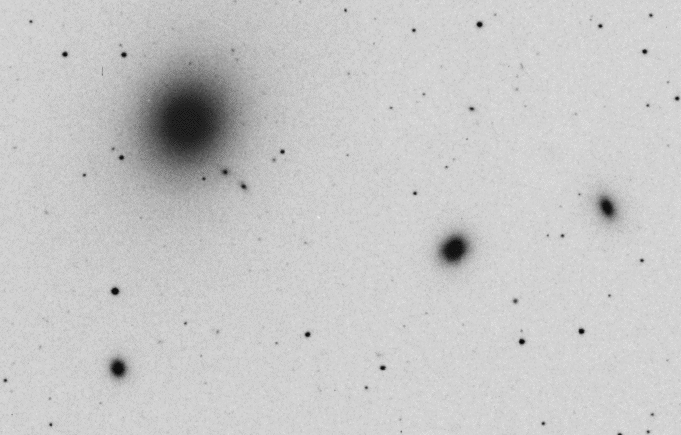
1. (5 pts) Distances to Clusters of Galaxies
We often see galaxies in groups. These galaxy clusters, analogous to star clusters, are groups of galaxies in the same region of 3D space. The following are pictures of 3 clusters of galaxies named Virgo, Coma, and Hercules, taken from the Digitized Sky Survey of the Space Telescope Science Institute. These clusters were named for the part of our sky (stellar constellation) that they appear in, although they are outside of and extremely far away from our own galaxy. The pictures are presented in "reverse video" meaning that what would look bright on the sky, like stars and galaxies, are shown here in black so that you can see details more clearly. The objects with spikes coming out of them are foreground (Milky Way) stars: anything that looks fuzzy is a galaxy.

(a)
(b)

(c)
(i) Place the three above clusters in order based on how far away they are from the Milky Way.
       A                 C                  B
    closest            middle             farthest
(ii) Explain on what galaxy cluster characteristics you made this decision.

(i) Sizes of the galaxies -- galaxies appear larger if closer
(ii) How many galaxies are visible in the image -- can see more in field of view if farther away.
(iii) Distances between galaxies -- the farther apart they appear to be the closer they are, if their actual distance is similar

2. Velocity - Distance Plot
(i) (3 pts) Plot the data from the table below to make a velocity-distance, Hubble Diagram for galaxy clusters.

      Galaxy Cluster     Velocity     Distance
                         (km/sec)     (Million LY)
      Virgo               1,200            39
      Ursa Major         15,000           500
      Corona Borealis    22,000           700
      Bootes             39,000         1,250
      Hydra              61,000         1,980

Use this diagram to answer the following questions.
(ii) (4 pts) From this plot determine the Hubble constant H. Remember Hubble's Law:
Velocity = Hubble Constant x Distance

H = V/D = 30.7, 30.0, 31.4, 31.2, 30.8 km/s/MLY

Average: H = average V/D = 30.8 km/s/MLY

(iii) (5 pts) From H calculate the age of the universe.
If (in our mind) we reverse the outward expansion of all the galaxy clusters and "run the movie backwards," we can determine the age of the Universe as the time when all these galaxies were in the same place. From Hubble's Law and the relation between velocity, distance and time, V=D/t or D=Vt, determine the time it took for the galaxies to get to where they are now. This is the maximum age of the universe. Derive the algebraic expression for this age of the universe, and then calculate the universe's age (in billions of years) (1 year = 3.14 x 107 sec).

V = HD = D/t

so time to reach a separation D going at velocity V is

t = D/V = 1/H

Must be careful of units. The easiest way is to convert the distances of the clusters from MLY to km

1 LY = 9.5x1012 km, so 1 MLY = 9.5x1018 km

      Galaxy Cluster     Velocity     Distance      Distance
                         (km/sec)     (MLY)         (km)
      Virgo               1,200            39       3.71x1020
      Ursa Major         15,000           500       4.75x1021
      Corona Borealis    22,000           700       6.65x1021
      Bootes             39,000         1,250       1.19x1022
      Hydra              61,000         1,980       1.88x1022

Then from D = V*t get t = D/V:

      t (Virgo)    = 3.71x1020 / 1,200  = 3.09x1017 sec
      t (Ursa Maj) = 4.75x1021 / 15,000 = 3.17x1017 sec
      t (Cor Bor)  = 6.65x1021 / 22,000 = 3.02x1017 sec
      t (Bootes)   = 1.19x1022 / 39,000 = 3.05x1017 sec
      t (Hydra)    = 1.88x1022 / 61,000 = 3.08x1017 sec
Average and convert seconds to years.

t = 3.08x1017 sec = 3.08x1017 / 3.14x107 = 9.8x109 years.

3. (3 pts) Which type of Galaxy has a stellar component most like the Nuclear Bulge of the Milky Way?
(a) An elliptical galaxy.
(b) An irregular galaxy.
(c) The disk part of a spiral galaxy.
This question was inadvertantly repeated from homework 9: Delete it.

4. (4 pts) Peculiar Galaxies
What is the primary evidence for there being a super-massive black hole in the center of an Active Galactic Nucleii? This question was inadvertantly repeated from homework 9: Delete it.

5. (5 pts) Look at the DEEP Image's Table at http://www.ifa.hawaii.edu/~cowie/k_table.html for the six objects with the largest redshifts (and thus farthest away from us and farthest back in time). How do these galaxies differ, in general, from the galaxies in Cluster A, problem 1? [Hint: the galaxy type is usually given in the last column, where 0=elliptical, 1=spiral, and 2=irregular.]

6 largest redshift objects (from spectra) are:

    Z      Type
    2.85   Spiral
    2.80   Irregular
    2.67   Spiral
    2.23   Spiral
    1.36   Spiral
    1.24   Elliptical

The 6 largest redshift objects (from 6 color photometry) are:

    Z      Type
    3.07   Spiral
    3.02   Irregular
    3.00   Irregular
    3.00   Irregular
    3.00   Irregular
    3.00   Irregular

At the largest redshifts irregulars and spirals dominate. In the cluster pictures earlier in this homework ellipticals dominated.

6. (3 pts) Number the following stages in the Big Bang evolution of the universe in their proper time sequence from beginning (1) to end (5).

                   _5_ galaxy formation

                   _1_ inflation 

                   _2_ primordial nucleosynthesis
 
                   _3_ radiation era 

                   _4_ recombination
7. (3 pts) Choose the best description of the distribution of galaxies in the Universe.
  1. clumped like Swiss cheese
  2. clumped in clusters in thin shells around voids
  3. clumped in uniformly distributed clusters
  4. clumped in randomly distributed clusters
  5. Limburger cheese
8. (3 pts) Because the universe is expanding, in the past the temperature of the matter and radiation in it was
  1. hotter than now
  2. the same as now
  3. cooler than now
Visions of the Universe
Beth Hufnagel's home page, email: hufnage4@pilot.msu.edu
Bob Stein's home page , email: steinr@pilot.msu.edu