





|

Electric
fields for simple geometries
Gauss's law
can derive the E-field for the following geometries:
A
spherically symmetric charge distribution,
e.g. a point charge.
The Gaussian surface should be a sphere. The area
of the surface is 4pr2, so
Gauss's law becomes:
where Q(r) is the charge inside the
surface. For a point charge, Q(r) is the
total charge, whereas for a uniform charge
density r (charge per unit volume) the
charge inside the surface is:
Thus for a uniform sphere of charge, the electric
field is zero at r=0, and grows linearly
in r inside the sphere, while outside
the sphere the electric field is of the same form
as Coulomb's law.
A
large plate with uniform charge density.
Consider the plate below, which we will assume is
infininte ( a good assumption if you are close to
the plate) and has a charge per unit area,
s.
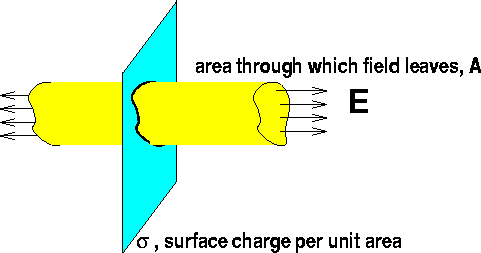
Gauss's law
states (remember the field leaves through both
sides):
Two
oppositely charged parallel plates.
If there were two plates with opposite charge,
the electric field would be double this value in
between the plates, 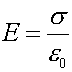 ,and zero
outside the plates. ,and zero
outside the plates.
An
infinite line charge.
Drawing a cylinder of length L around an
infinite wire, which has a charge per unit length
of l, will trap a charge inside
of Q=lL, and the area
through which the flux leaves is 2prL.
Gauss's law then states:
Examples
Gauss's law index
|