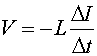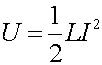Inductance

The Inductance L is a measure of the voltage drop across an iductor (e.g. a solenoid) per the rate change of current.

Since the magnetic field inside a solenoid is proportional to a current, the rate-change of the magnetic flux is proportional to the rate-change of the current. From a previous lecture, the magnetic field inside a solenoid was given as:

The voltage across the solenoid is given by the rate-change of the flux times the number of loops:

Therefore, the inductance L can be given in terms of the geometry of the solenoid.

Longer solenoids and solenoids with more cross-sectional area have more inductance. The strongest dependence is on the number of turns per unit length. One can also increase the inductance by using iron instead of a vacuum. One would replace the parameter m₀ with m if the material had a different susceptiblity.

We have now considered three circuit elements (aside from batteries). The voltage across each element depends on a different aspect of the charge.

1. Capacitors: V = (1/C) Q
   The voltage drop across the capacitor is proportional to the charge on the capacitor.

2. Resistors: V = RI
   The voltage drop is proportional to the current, which is the rate-change of the charge.

3. Inductors: V = L (DI/Dt)
   The voltage drop across the inductor is proportional to the rate-change of the current.

A resistor keeps the charge from changing instantly in a circuit, because an instant change in the charge constitutes an infinite current which implies an infinite voltage. Similarly, an inductor keeps the current from changing suddenly. Unlike a capacitor, current can continuously flow through an inductor.

An inductor can also store energy. The power being stored in an inductor is:

A little calculus would show that the energy stored in an inductor is:

Examples     Induction's main page