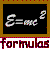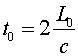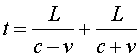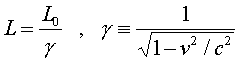Length contraction Moving objects appear shorter in the dimension parallel to their velocity, again by the g factor introduced previously. To derive the contraction we again consider a light clock as the case of time dilation, only in this case we consider the clock on its side such that the motion of the clock pulse is parallel to the clock's velocity. If the clock has length L0 in the rest frame of the clock, the time to for light to bounce from one side of the clock and back is:
However to an observer who sees the clock pass by with a velocity v, the light appears to take more time to traverse the length of the clock when the pulse is traveling in the same direction as the clock, and it appears that it takes less time for the return trip.
We know from the time dilation that
Therefore the above three equation can be used to eliminate t and t0 to obtain the result
Thus moving meter sticks appear shorter along the direction of motion. |