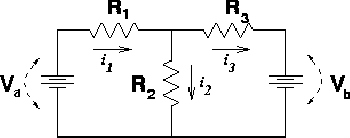
Kirchoff's laws / RC Circuits Examples
Example #1
Problem:
Find the currents through all the resistors in the circuit below:

DATA: Va = 12 V, Vb = 12 V, R1 = 10 W, R2 = 15 W, R3 = 20 W
Solution:
Summing the voltages around the left and right loops gives the following two equations:
![]()
![]()
![]()
One can then reduce the problem to "2 equations 2 unknowns" by substituting for i1 and obtaining 2 new equations.
One can add these two equations together to eliminate the the i3 term since all three resistors are the same, which will be now noted by R.
![]()
Once i2 is known, Eq. (4) can be used to get i3 and i1 can be found by summing i2 + i3.
i2 = 0.554 amps, i1= .369 amps, i3 = -.185 amps
Example #2
Problem:
Find the charges on all the capacitors in the circuit below:

DATA: Vb = 12 V, C1 = 10 mF, C2 = 15 mF, C3 = 20 mF
Solution:
Summing the voltages around the left and right loops gives the following two equations
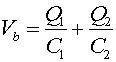
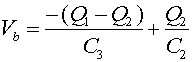
where Q3 has been replaced by Q1 - Q2. Dividing Eq. (1) by C3, dividiing Eq. (2) by C1, then adding the equations yields:

which rearranged yields
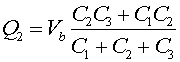
Once Q2 is known, Eq. (1) can be used to get Q1, and Q3 can be found as the difference Q1 - Q2.
Q2 = 120.0 mC, Q1= 40.0 mC, Q3 = -80 mC
Example #3
The circuit below has been in position a for a long time. At time t = 0 the switch is thrown to position b. DATA: Vb = 12 V, C = 10 mF, R = 20 W
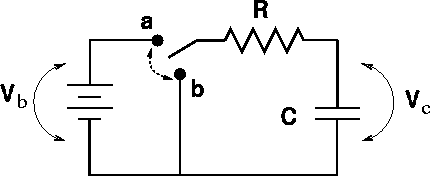
a.) What is the curnent through the resistor just BEFORE the switch is thrown?
I = 0
b.) What is the current through the resistor just AFTER the switch is thrown?
Solution: I = V/R
I = 0.6 amps
c.) What is the charge across the capacitor just BEFORE the switch is thrown?
Solution: Q = CV
Q = 120 mC
d.) What is the charge on the capacitor just AFTER the switch is thrown?
Solution: Charge does not change instantaneously.
Q = 120 mC
e.) What is the charge on the capacitor at at time t = 0.3 msec after the switch is thrown?
Solution: Q = Q0exp(-t/t) , where t = RC = 0.2 msec
Q = 26.8 mC
Example #4
Considering the same circuit, only with the switch thrown from b to a at time t = 0 after having been in position b for a long time. DATA: Vb = 12 V, C = 10 mF, R = 20 W

a.) What is the curnent through the resistor just BEFORE the switch is thrown?
I = 0
b.) What is the current through the resistor just AFTER the switch is thrown?
Solution: I = V/R
I = 0.6 amps
c.) What is the charge across the capacitor just BEFORE the switch is thrown?
Solution: Q = CV
Q = 0
d.) What is the charge on the capacitor just AFTER the switch is thrown?
Solution: Charge does not change instantaneously.
Q = 0
e.) What is the charge on the capacitor at at time t = 0.3 msec after the switch is thrown?
Solution: Q = Q0(1.0 - exp(-t/t)) , where t = RC = 0.2 msec
Q = 93.2 mC