Not only has astronomy been humanity's timekeeper or chronologer but it has also paid its way by providing a system of navigation. Ships at sea, camel caravans on the trackless desert, and aircraft on long journeys all found their way by sighting celestial objects.
Finding your way by the stars, celestial navigation as it is called, makes use of two systems of coordinates. One, the celestial coordinate system, marks the positions of the stars on the sky. The other, the geographical coordinate system (the familiar longitude-latitude system) marks the positions of places on Earth.
If the Earth did not rotate--celestial navigation would be easy, for a particular region of the sky would always lie over the same region on the surface of the Earth. Thus, recognizing the region of the sky that is overhead would immediately indicate position on Earth. However, the Earth does rotate, and different regions of the sky pass over any particular region on Earth at different times.
So the fact that the Earth rotates makes it necessary to include time measurements in the linking of the celestial and geographical coordinate systems. The necessity was well understood by sailors on long ocean journeys in the days prior to radio communications. Errors in navigation could, and did, lead to shipwreck and loss of life. One such tragedy, a British naval disaster that occurred near the Scilly Isles in 1707, cost the lives of about 2000 sailors
Spurred by the tragedy, Sir Isaac Newton, then a member of the British Parliament, suggested that prizes with a top award of 30,000 pounds (then a considerable fortune) be given to someone who could, by whatever method, determine longitude at sea within 1 degree, or 60 nautical miles (111 kilometers). A "Board of Longitude" was appointed to make an award when a majority of the commissioners were convinced that a proposed method was practical and the method was proven accurate to within the prescribed limits on an ocean voyage from Britain to the West Indies.
An Englishman, John Harrison, attacked the problem of finding longitude at sea by building very accurate clocks. As an alternate method, a German astronomer, Tobias Mayer, constructed tables of the positions of the moon for various times. His tables were based on the very accurate gravitational analysis of the moon's motion that had been made by the great Swiss mathematician Leonhard Euler.
After years of tests and squabbles between Board of Longitude, astronomers, Parliament, the admiralty and other mariners, Harrison's method was shown to be the more accurate, within one-half degree of longitude; but Mayer's method was shown to be sufficiently accurate and more practical. In fact, Mayer's method was adopted by most ships' captains.
In 1765 the board decided to make partial awards to Harrison and to Mayer, the latter's award being posthumous. However, Harrison had valid claim to the top prize; and in 1773, upon the intervention of King George III, he received the remaining amount of his prize.
Time, navigation, coordinate systems--these are, in fact, concepts which have been formalized within the framework of astronomy. These concepts deal with the most basic aspects of astronomy, that is, with the shape of the Earth and its motions. The Earth's motions determine the coordinate system that we use to locate the stars in the sky.
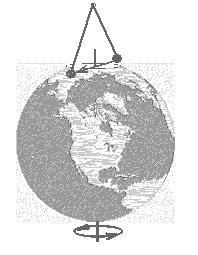
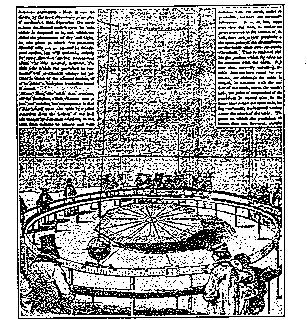
There are three major motions of the Earth: rotation on its axis, revolution about the sun, and precession. Apart from observing its rotation from a space vehicle, the Earth can be proven to rotate by means of a special kind of pendulum; a massive weight suspended on a long steel wire which, in turn, is attached to a frictionless pivot. The pendulum is therefore able to swing in any direction. If, as shown in Figure 1, such a pendulum were placed at the North Pole and set in motion toward a distant star, the plane of its swing would remain fixed relative to the star, but it would appear to rotate relative to the ground. In reality, the plane of swing is fixed in space, and it is the Earth that turns beneath it. Newtonian mechanics predicts a similar but slower apparent rotation of the plane of swing relative to the ground when the pendulum is placed away from the pole and closer to the equator. At the equator there is no apparent rotation of the plane of swing. This type of pendulum is frequently seen in museums of science and technology. It is one of the most easily demonstrated proofs that the Earth rotates and is called a Foucault pendulum after its inventor. The first public proofs that the Earth rotates and is called a Foucault pendulum after its inventor. The first public demonstration of this phenomenon was given by Professor J.B.L. Foucault in 1851 under the dome of the Pantheon in Paris, using a wire 200 feet in length with a 60 pound weight attached.
 |
 |
FIGURE 1 (left) Foucault Pendulum at the North Pole. The plane of the pendulums swing stays fixed while the Earth turns beneath it. (right) Illustration of the original Foucault experiment from a 1851 newspaper.
The revolution of the Earth about the sun can be proven by the observation of stellar parallax or stellar aberration. Parallax is the angular change in the line of sight to a nearby star as it is viewed from different points in the Earth's orbit. The effect is very small and was not observed until 1838. More than 100 years earlier, James Bradley, while trying to detect parallax, discovered the phenomenon of stellar aberration. He observed that all stars show an annual angular motion about their average positions. The maximum displacement is roughly 20.5 seconds of arc. Aberration is accepted as direct observational proof of the Earth's orbital motion and the finite speed of light. It is best explained by an analogy with falling rain.
Imagine standing in a gentle rainfall on a windless day. For maximum protection, you would hold your umbrella vertically. But if you start to run rapidly through the rain, you will now want to tip the umbrella in the direction in which you are running, because the apparent motion of the raindrops now becomes a combination of your motion and the natural descent of the raindrops.
Since the speed of light is not infinite, the apparent direction from which it comes will also be influenced by the motion of the observer. As shown in Figure 2, the continual change in the direction of orbital motion of the Earth causes an observer to adjust the direction of the telescope continuously to agree with the apparent direction of the starlight. The precise correction in the direction depends upon the position of the star in the sky and the direction of the Earth's motion at that moment. The latter, of course, depends upon the time of year when the observation is being made.
The third of the Earth's major motions, precession, is the one that appears the most mysterious. It can also be explained by the application of Newtonian mechanics. Because of its rapid rotation, the Earth is deformed from a perfect sphere and has a slight bulge at the equator. The deformation is very small; the equatorial diameter of the Earth becoming only one-third percent larger than its polar diameter; but it is still enough to cause the observed precession. The plane of the Earth's equator is inclined at 23½ degrees to the plane of the Earth's orbit. The sun is always in the plane of the Earth's orbit, and the moon is never far away from that plane. Because the attraction of the sun and moon on the Earth's bulge is slightly greater for the side which is nearer to them than for the far side, their combined attraction, on the average, attempts to pull the plane of the Earth's equator into agreement with the plane of the Earth's orbit. In other words, they act to try to reduce the 23½-degree angle. This is schematically illustrated for the moon in Figure 3, where the moon is shown near the point in its orbit at greatest southerly distance from the plane of the Earth's equator. Since the distance of the sun and moon from the plane of the Earths equator continually changes, the turning forces that they apply to the Earths bulge continually change, on a semi-annual basis for the sun and semi-monthly for the moon.
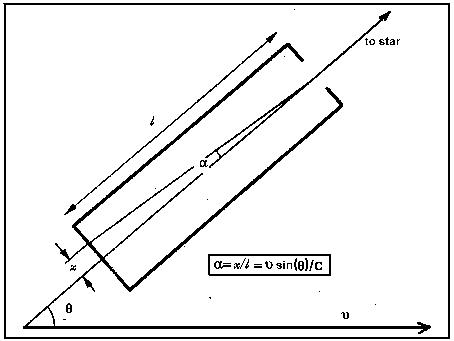 |
FIGURE 2 Stellar aberration. In order to keep a telescope pointed at starlight arriving perpendicular to the Earth's orbit, the telescope must be tipped in the direction of motion of the Earth in its orbit. The tilt angle is given in the box above, since the earth moves by the distance x during the time it takes light to travel down the telescope tube. [where v is the velocity of the earth in its orbit and c is the velocity of light].
The application of Newtonian mechanics shows that when an attempt is made
to turn a rapidly spinning body about an axis different from its axis of
spin, the body turns instead about a third axis. This third axis is always
perpendicular to both the spin axis and the axis about which the attempted
rotation occurs. But, as the body moves about the third axis, the direction
in which the spin axis points, and therefore the third axis (or the so-called
axis of precession), also changes because it must always be perpendicular
to the spin and turning axis. The net result is that the spin axis undergoes
a conical motion in space. A toy top is a good example of this phenomenon.
The force of gravity acts to cause the top to fall over on its side. Instead
of falling over, however, the top's spin axis precesses in a cone about
the vertical direction until the top stops spinning--then it falls over.
The Earth has a similar motion due to the average influence of the sun and moon upon its rotational bulge. The Earth's axis makes a cone in space, maintaining its tilt of 23½ degrees with the perpendicular to its orbit. The direction of motion is westward; takes the Earth roughly 26,000 years to make one complete conical motion. Because the influence of both the sun and moon varies throughout the year and the month, there is a slight wobble introduced into the conical motion known as nutation. This causes small periodic variations in the 23½-degree angle and in the westward movement of the Earth's axis of rotation.
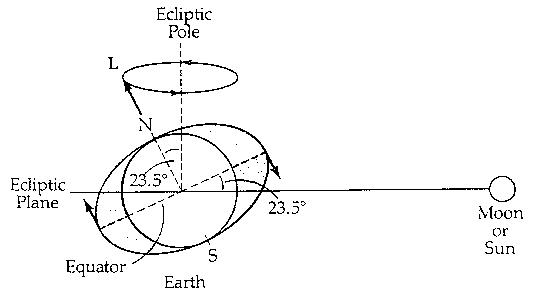 |
FIGURE 3 The gravitational influence of the moon upon the rotational bulge of the Earth. The stronger attraction of the moon on the nearby side of the Earth's bulge relative to that on the far side results in 2 turning force which tries to reduce the obliquity of the ecliptic.
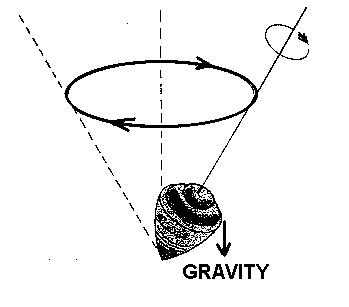 |
FIGURE 4 A comparison of a top's precession and the Earth's precession. The force of gravity on the top acts to make the angle of its cone of precession larger. The forces on the Earth's rotational bulge act to make the angle of the Earth's cone of precession smaller.
Note in Figure 4 that the top and the Earth rotate, or spin, in the same direction, but they precess in opposite directions. This is because gravity tries to make the top fall over; that is, it tries to cause the angle of the cone of precession of the top to increase. For the Earth, however, the forces of the sun and moon upon the Earth's rotational bulge try to decrease the 23½-degree angle of the cone of the Earth's precession. The direction of precession of a spinning body depends upon both the direction of spin and the direction in which the forces that give rise to the precession are being applied. Hence, the reversal in the turning forces between the top and the Earth results in the reversal of their precessions.
As the Earth's axis moves westward in its conical motion, the vernal equinox, which marks one of the intersections of the equator with the ecliptic, must also drift westward. Hipparchus, the ancient Greek astronomer, had discovered this slow, westward precession of the equinoxes around 120 B.C. Its cause, however, was to remain unknown for more than 18 centuries until Isaac Newton gave the physical explanation of why the equinoxes move.
The changing appearance of the sky with the observer's changing position confirms that the Earth is essentially spherical in shape and leads to a system for locating positions on its surface. As we have seen, it is the Earth that actually rotates in the eastward direction. The apparent westward rotation of the sky is merely a reflection of the Earth's motion, The two points on the Earth's surface in the line joining the celestial poles are called the poles of the Earth's rotation. The circle on the Earth midway between these points is the equator of the Earth. The distance of a location from the equator toward either of the Earth's poles can be measured by finding the angle from the equator to the line joining that location and the center of the Earth. This angle is called the geographical latitude of the location. All points at the same latitude will lie on a circle parallel to the equator called a parallel of latitude.
Because of the obvious difficulties of measuring the angle directly, we pass a circle through the poles of the Earth and the location of interest. The latitude of the point is then the fraction of the circle between the point and the equator expressed in degrees. The value of the latitude must lie between 0 and 90 degrees, since the pole, the farthest point from the equator, lies one-quarter of a circle away from the equator. The circle, through the point along which its latitude is measured, is called a meridian of longitude. The latitude of a point is called North if it lies closer to the North Pole than to the South Pole and South if the reverse is true.
The location of a place along its parallel of latitude is found by comparing its meridian of longitude with the meridian of longitude through Greenwich, England, which is called the prime meridian. The geographical longitude of a point on the Earth's surface is the fraction of a circle that is intercepted on the equator by the prime meridian and the meridian of longitude of the point. Longitude is measured from the prime meridian from 0 to 180 degrees eastward in the direction of the Earth's rotation and 0 to 180 degrees westward in the opposite direction. Thus, the two arcs indicated in Figure 5 uniquely locates Washington, D.C., that has coordinates longitude 77 W and latitude 38 N.
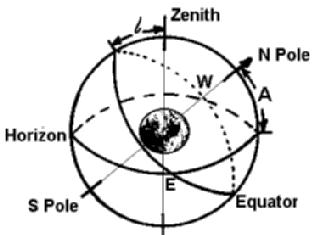
This description of latitude and longitude is easy to apply to a globe representing the Earth. On the actual Earth, the determination of latitude and longitude requires the use of the apparent celestial sphere. First, consider latitude. As shown in Figure 6a, for an observer positioned at the equator, the zenith lies on the celestial equator. Therefore, the North Celestial Pole lies on the Horizon; or, to put it another way, the altitude of the pole is zero degrees. As the observer moves toward the pole, as shown in Figure 6b, the zenith moves toward the celestial pole by the same angle that the observer has moved in latitude. The northern horizon, therefore, appears to dip below the pole by this same amount. In other words, the altitude of the pole is equal to the latitude of the observer. The basic task of a surveyor in measuring a latitude is consequently the measurement of the altitude of the celestial pole seen from that location. A latitude determined in this way is called an astronomical latitude and usually differs somewhat from the latitude given on a map because of slight irregularities in the gravitational field and the shape of the Earth.
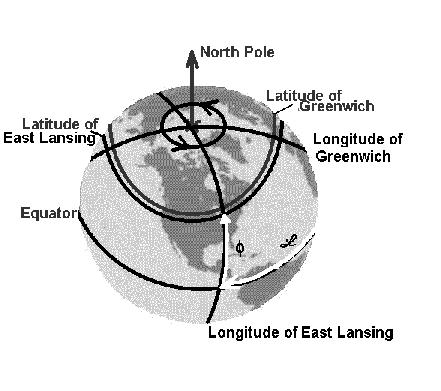 |
FIGURE 5 The longitude and latitude system. Longitude is measured east or west along the equator from the prime meridian. Latitude is measured north or south from the equator.
Longitude is somewhat more difficult to measure, although the measurement is, in principle, simple. Begin by identifying the observer's celestial meridian, the circle passing through the celestial meridian, and the observer's zenith. The celestial meridian lies directly over the observer's meridian of longitude. Consider another observer at Greenwich, where the celestial meridian of Greenwich corresponds to the prime meridian of longitude. Assume a star to be on the celestial meridian of Greenwich, as shown in Figure 7. Then, since the Earth rotates eastward once in 24 hours, the meridian of an observer to the west of Greenwich will advance toward the same star at a rate of 15 degrees each hour. Timing how long it takes the star to reach the observer's meridian after it has crossed that of Greenwich is, thus, equivalent to measuring the longitude from Greenwich to the observer. The longitude for this situation would be labeled West. If the observer had been in the Eastern Hemisphere, the star, naturally, would cross the observer's meridian before it crossed that of Greenwich. The time interval converted to degrees at the rate of 15 degrees per hour would still be the longitude. The longitude would be labeled East in this case, however. Frequently the longitude is expressed as the time difference between when a star crosses the meridian of Greenwich, and when it crosses the meridian of the observer, without converting to degrees. Longitude determination requires a precise knowledge of time and led to the development of accurate clocks to permit navigation at sea.
 |
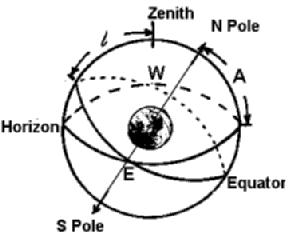 |
FIGURE 6 The change in the celestial pole and the celestial equator as the observer changes latitude. The figure on the left shows a position at 40 degrees latitude (l = A = 40) and that on the right shows one at 60 degrees. The observer's zenith moves from the celestial equator toward the celestial pole as the observer moves from zero latitude toward higher latitude.
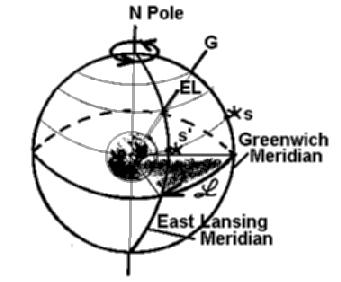 |
FIGURE 7 The determination of longitude by timing star transits. The difference in the times of transit of a star on the celestial meridian of Greenwich (at G) and the observer's meridian (at EL) is equal to the observer's longitude. The longitude is east if the star crosses the observer's meridian first and west if it crosses the meridian of Greenwich first. In this case the star crosses at s before it crosses at s'. (i.e. East Lansing is West of Greenwich.)
The technique for locating objects on the Earth may be applied to locating stars on the celestial sphere. The celestial equator is now the important circle. The angle between the equator and the direction of a star is called the declination of the star; it is usually symbolized by d or Dec. Conceptually, declination is analogous to geographical latitude. Since a star keeps the same declination throughout a day, its diurnal circle is also a parallel of declination. All other stars with the same declination lie on the same parallel. To locate a given star on its parallel, the celestial meridian can be used in much the same way that the meridian of Greenwich is used on Earth. Pass a great circle through the celestial poles and the given star. This circle is called the hour circle of the star. The angle at the equator between the celestial meridian and the hour circle of the star is called the local hour angle (LHA) of the star. It is always measured westward from 0 through 360 degrees or 0 through 24 hours. Again, 1 hour of angle equals 15 degrees of angle. When a star has an hour angle of 0, it is highest in the sky on the observer's meridian and is said to be in transit (or more properly, upper transit). The precise measuring scheme is shown in Figure 8. Notice that since the sky appears to move westward, the local hour angle of the star is continually changing. For some purposes this is a nuisance; but for timekeeping, it proves to be very helpful.
For cataloguing the positions of stars, it is desirable to have a coordinate system fixed to the stars, where both coordinates are unchanging, rather than a system fixed to the observer, who is actually in motion because of the Earth's rotation. Declination can be used as before because a star's declination does not change. The coordinate measured along the celestial equator can also be fixed by using a fixed point on the equator from which to measure. The point of reference which is chosen is the vernal equinox, indicated by the sign of Aries the Ram, ^. The vernal equinox is the point where the ecliptic (the sun's annual apparent path) cuts the equator such that a body traveling eastward on the ecliptic would pass from the southern to the Northern Hemisphere of the sky. The right ascension of a star is defined as the angle along the equator from the vernal equinox to the circle through the celestial poles which also passes through the star. The manner in which the right ascension-declination system is used and demonstrated in Figure 9. Right ascension, symbolized by a or RA, is always measured eastward from the vernal equinox, ranging in value from 0 through 24 hours.
 |
FIGURE 8 The declination-hour angle coordinate system. The local hour angle (LHA) is measured westward from the observer's meridian along the celestial equator. Declination (d) is measured North or South from the celestial equator.
Using the right ascension-declination, or equatorial, system, maps of the sky can be drawn similar to the one depicted in Figure 10. Right ascension is always shown in the horizontal direction, increasing eastward to the left, and declination is always shown in the vertical direction, positive northward and negative southward. Due to precession, the celestial poles continually move relative to the stars. Hence, the celestial equator must also move, causing the vernal equinox to move westward slowly along the ecliptic. Because of this motion, the right ascension and declination of a star are continually changing, but very slowly. It is necessary to update the position of a star for the precession of the equinoxes. This is a nuisance requiring a rather straightforward calculation when the coordinates of a star are needed for a certain night, but its position has been given on a map, or in a catalogue of positions, for a different time.
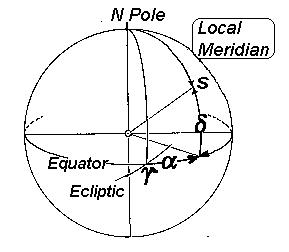 |
FIGURE 9 The declination (d) is measured N and S from the equator and the right ascension (a) is measured eastward from the vernal equinox (Y) along the celestial equator |
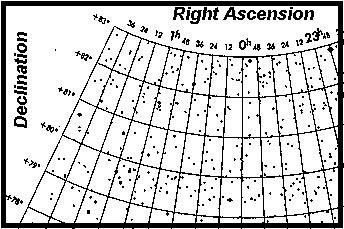 |
FIGURE 10 A map of part of the celestial sphere.
The hour angle system can now be used to help us keep time. Local sidereal time, or star time, is defined as being equal to the local hour angle of the vernal equinox. The word local is required because this time is measured in reference to the observer's, hence the local, celestial meridian. Unfortunately, the vernal equinox is not visibly marked on the sky. We know that if the local sidereal time has a particular value, the vernal equinox is west of the observer's meridian by that hour angle. Alternately, the observers meridian is eastward of the vernal equinox by that same angle. But the angle measured eastward from the vernal equinox is right ascension. Therefore, as shown in Figure 11, the local sidereal time is equal to the right ascension of a star in transit at the given instant. A telescope called a meridian transit is used to determine sidereal time. This instrument has a horizontal axis that is aligned due East and West. As the telescope is rotated on its axis, its field of view sweeps along the observer's celestial meridian. If the telescope is pointed at the declination of a particular star, the observatory sidereal clock can be synchronized with the Earth's rotation by noting that the clock should read a time equal to the right ascension of the star when the star is precisely in the center of the field of the telescope. Sidereal time is useful for finding the positions of stars in the sky, but not for everyday use. For this purpose we prefer to tell time by the sun. Due to the Earth's orbital motion around it, the sun appears to move around the ecliptic once each year; thus, it is continually changing its position relative to the vernal equinox. In general, sidereal noon, when the vernal equinox transits the meridian, does not coincide with solar noon, when the sun transits the meridian. In other words, during the year solar noon occurs at progressively changing times as measured by the sidereal clock.
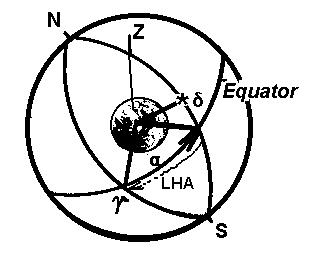 |
FIGURE 11 Determination of local sidereal time by star transits. The local hour angle of the vernal equinox (that is, local sidereal time) equals the right ascension of a star upper transit.
The time used for our daily living is synchronized with the sun. Local apparent solar time equals the local hour angle of the apparent sun plus twelve hours. The twelve hours are added to make the solar day start at midnight rather than at noon. The apparent sun is simply the sun as we see it in the sky. The measurement of apparent solar time is illustrated in Figure 12. To estimate the difference between the solar and the sidereal day, refer to Figure 13.
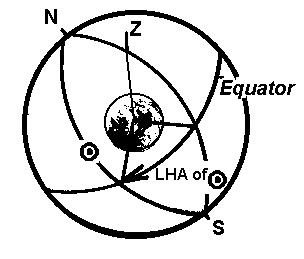 |
FIGURE 12 Local apparent solar time. The local hour angle of the apparent sun plus 12 hours equals the local apparent solar time.
Assume the Earth to be at the point in its orbit where sidereal and solar noon happen to occur together. One sidereal day later the Earth will have made one full rotation relative to the vernal equinox; but it will also have advanced nearly one degree in its orbit during that time. Therefore, it will have to rotate approximately one degree more to bring the sun back to the observer's meridian. It takes the Earth nearly 4 minutes to rotate through 1 degree. Therefore, an interval of 24 hours of solar time is roughly 4 minutes longer than 24 hours of sidereal time. A solar clock consequently appears, to run slower than a sidereal clock, "losing" 4 minutes each day.
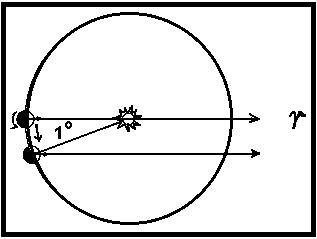 |
FIGURE 13 Comparison of the solar and the sidereal day. The annual eastward motion of the sun in the sky causes it to move approximately one degree per day. This is the approximate additional amount over 360 degrees by which the Earth must rotate to bring the sun to two successive transits. It takes the Earth roughly four minutes to rotate one degree.
 |
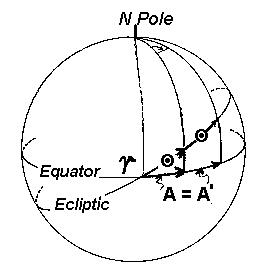 |
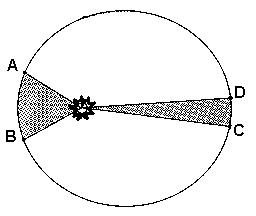
FIGURE 14 Causes of the variations in the length of the apparent solar day. (left) The eccentricity of the Earths orbit (exaggerated for clarity) causes the sun's apparent motion through the stars to vary. (right) Because of the obliquity of the ecliptic, to produce the same change in right ascension (A = A'), the sun must travel further along the ecliptic when it is far from the equator than when it is near the equator.
The best way to tell apparent solar time is with a sundial. Until mechanical clocks were invented, a sundial was the only good way of keeping time. However, mechanical clocks do not keep exact pace with the sundial, not because the clocks are bad, but because the apparent solar day varies in length throughout the year. The two causes of this variation are illustrated in Figure 14. First, the Earth's orbit is elliptical. The Earth therefore speeds up and slows down in its orbital motion in accord with Kepler's law of equal areas. Thus, the apparent solar day is longer when the Earth is at perihelion, and moving fastest, than when it is at aphelion, and moving slowest. The second cause of the changing length of the apparent solar day results from the obliquity of the ecliptic. Because of this 23½-degree angle between the ecliptic and the equator, a movement of the sun, when farthest from the equator, causes a greater change in the right ascension of the sun than an equivalent movement of the sun when near the equator. Since time is reckoned relative to the equator, this difference introduces a further variation in the length of the apparent solar day. To circumvent this problem, the concept of the mean sun is introduced. The mean sun is a fictitious sun that moves uniformly along the celestial equator. It goes once around the equator at constant speed each time the real sun appears to go once around the ecliptic. Local mean solar time, schematically depicted in Figure 15, is equal to the local hour angle of the mean sun plus 12 hours. Since the mean sun is fictitious, it is not directly observable. However, from a knowledge of the Earth's orbital motion, the position of the mean sun relative to the vernal equinox can be calculated. The position of the vernal equinox can be found by observing transits of the stars. Consequently, solar clocks are regulated at observatories by timing transits of stars. The sidereal time so observed is transformed to mean solar time by calculation; this time, in turn, is used to check a mean solar clock.
 |
FIGURE 15 Local mean solar time. The local mean solar time is equal to the local hour angle of the mean sun plus 12 hours.
Local mean solar time changes as you move eastward or westward in longitude. In fact, the difference in reading between two mean solar clocks at two places will be exactly the difference in the longitudes of the two locations. Strictly speaking, whenever you move eastward or westward, you should reset your local clock, no matter how little your longitude has changed. To remove this nuisance, standard time zones have been established. By common agreement all clocks within a time zone are set to read the same, that is, to keep standard time. The standard meridians of each zone differ by integer multiples of 15 degrees (or 1 hour) in longitude from the meridian of Greenwich. The standard zones, therefore, always differ by an exact number of hours from the time at Greenwich. Notice in Figure 16 how the zone boundaries have been adjusted to conform to geographical and political boundaries. The numbers at the top of the diagram indicate the number of hours that must be added to that particular standard time in order to find Greenwich time. Greenwich time, by the way, is frequently called universal time; it is the time used in tabulation of time occurrence of astronomical events. Daylight saving time is simply the time that would be kept at tile next standard meridian East of the normal meridian for a particular zone. Clocks are therefore advanced one hour, which makes sunset occur one hour later by tile clock and gives the appearance of adding one more hour of daylight in the evening, hence its name. Of course, one hour of daylight is stolen from the morning. A convenient way to remember which way to change the clock when converting from standard to daylight saving time, or vice versa, is the saying "spring forward, fall back."
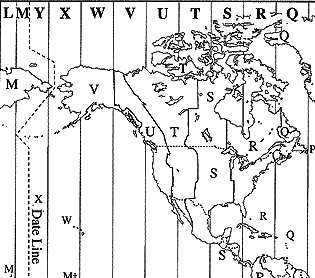 |
FIGURE 16. Standard time zones for North America. For political reasons
these do not follow true lines of constant longitude. (From The US Naval
Observatory Astronomical Applications Department Click
here for a world map)
The apparent solar day was the basis of timekeeping for much of the history of civilization. We still live by the sun, but we have improved on it as a keeper of precise time. This improvement did not start, however, until pendulum clocks were introduced in the last half of the seventeenth century and began to replace sundials as the means of determining time.
These first crude clocks were usually used to keep time between sundial readings because they were just not good enough to operate at a constant rate for long periods of time. It was not until the eighteenth century, when good mechanical clocks (often called chronometers) became available, that the first real break with apparent solar time was made and the concept of mean solar time began to take hold. Even into the early nineteenth century, it was still common practice to publish tables for observing the skies and for navigation in terms of apparent solar time. The length of the apparent solar day changes throughout the year, so the second, usually taken as the smallest basic unit of time, was not the same from day to day. But a well-designed clock keeps the same value of the second for extended periods of time, and it was this constancy that drove astronomers to look for a more uniform celestial timekeeper, the fictitious, mean sun. By the late eighteenth and early nineteenth centuries, mean solar time had come into general use.
Solar time is determined mainly by the Earth's rotation. Mean solar time simply averages out the speeding up and slowing down of the sun through the stars due to the characteristics of the Earth's orbit. Thus mean solar time is nothing more than a convenient way of measuring how much the Earth has turned on its axis and is certainly more convenient than sidereal time for everyday living purposes. A problem still remains, however, because the rotation rate of the Earth is not exactly constant, or, as we say relative to time, not precisely uniform.
The variable rotation of the Earth was apparent even as early as Newton's time, but it was not really scientifically determined until the beginning of the twentieth century. At that time it became well established, from studies of ancient eclipse records and the observations of planets, that celestial bodies appear to be moving ahead of where they should be in the sky. The celestial bodies are not at fault; it is our timekeeper, the Earth, that is slowing down. The day is increasing in length by roughly one thousandth of a second each century due to tidal friction caused primarily by the moon. The tides tend to remain aligned with the direction toward the moon. Since the Earth rotates eastward more rapidly than the orbital motion of the moon carries it eastward, the Earth literally spins beneath the tides. Friction between the solid surface of the Earth and the waters of the tides functions as a brake to slow the rotation of the Earth. In addition, with the development of crystal-controlled clocks, it had become possible to measure seasonal changes in the Earth's turning resulting from atmospheric and oceanic changes induced by seasonal changes in temperature. There are also abrupt, small irregular changes in the Earth's rotation period induced by seismic readjustments, of material within the Earth. It is even possible to correlate some of these occurrences with major earthquake events.
With these discoveries it became evident that a better unit of time was needed. In 1952 the International Astronomical Union, meeting in general assembly in Rome, adopted the concept of Ephemeris Time. This is a dynamical time; that is, it is the time required to make Newton's laws of motion predict the observed positions of the planets. The Ephemeris Second is tied to mean solar time by its definition, 1/31556925.975 of the length of the tropical year at the beginning of the year 1900. The correction that must be applied to Ephemeris Time to find mean solar time is in essence found after the fact by comparing the mean solar times of observations with the predicted positions of objects given in Ephemeris Time. The correction cannot be well determined until observations have been collected over two or three years, but our knowledge of past trends allows the estimation of an approximate value for a few years into the future. In 1980, the correction from Ephemeris Time to Universal Time (the mean solar time at Greenwich, England) had built up to 151.96 seconds from the correction of 3.90 seconds in 1900.
In the 1950's and 1960's a new type of clock was introduced, the so-called atomic clock. An example is shown in Figure 17. in these systems the fundamental frequencies associated with the structure of atoms are used to keep time. In 1972, by international agreement, the International Second (SI) was defined as the duration of 9,192,631,770 vibrations of the radiation corresponding to a particular spectral line of the isotope cesium-133. Cesium clocks are now in use at various national laboratories throughout the world. These laboratories report their results to the Bureau Internationale de I'Heure in Paris, France, where they are combined to form InterNational Atomic Time (TAI). This is converted to Mean Solar Time and, being given in terms of a clock at Greenwich, England, is called Coordinated Uni Time (UTC). In the United States, the National Bureau of Standards broadcasts time signals in UTC over radio station WWV (frequencies 5, 10, and 15 MHz). Whenever the difference between TAI and UTC accumulates to 0.9 second, a leap second is introduced in Universal Time to bring them back into agreement.
 |
FIGURE 17 A modern atomic clock (NIST-7), accurate to one part
in 5*10-15. (From A
History of the NIST Time and Frequency Division National Institute of Standards
and Technology by D.B. Sullivan, J.A. Wessels, Time and Frequency Division,
Boulder, CO
The next time someone asks you for the time. you should ask them which kind of time before responding.
Besides the day, there is a longer period that is used for timekeeping: the year, or the length of time it takes the sun to make one complete apparent path about the ecliptic. But, just as there are different kinds of days, there are different kinds of years, depending on the reference point by which one trip around the ecliptic is measured. We will be concerned with only two kinds, the sidereal year and the tropical year. The sidereal year is the interval between successive conjunctions of the sun with a particular star, and is the true period of the Earth's revolution about the sun. Strictly speaking, we should say a fixed direction in space instead of a particular star, because (as will be demonstrated later in the book) the stars are not really fixed. The tropical year is the interval between successive passages of the sun through the vernal equinox. Since the vernal equinox is moving slowly westward through the stars due to precession, the tropical year is slightly shorter than the sidereal year, as shown in Figure 17. The sidereal year is the true period of the Earth's revolution and is 365.2564 days in length, The tropical year is often called the year of the seasons; it is 365.242199 days in length.
Due to the phenomenon of the seasons, the tropical year was the first to be discovered, although it was only crudely determined. The seasons are a direct result of the obliquity of the ecliptic. Shown in Figure 18 is a plot of the sky showing the relationship of the ecliptic to the celestial equator. The two points of intersection of these circles are called the equinoxes, which means equal nights. When the sun is at either of these points, its declination is zero, and it therefore is above the horizon for 12 hours and below it for 12 hours. The two points of extreme distance from the equator are called the solstices, which means the sun comes to a stop. It does, in fact, stop its movement in declination since it must reverse its direction of movement in declination at these locations. It has a declination of 23½ºN at the summer solstice and 23½ºS at the Winter solstice. When the sun is not at an equinox, the length of time the sun is above the horizon is different from that for which it is below the horizon; the greatest difference occurs when the sun is a solstice. In the Northern Hemisphere there are more daylight hours than nighttime hours during the interval between the vernal and autumnal equinoxes. The opposite is true during the interval between the times of the autumnal and vernal equinoxes. To understand the seasons, consider Figure 19, which schematically represents the circumstances at the summer solstice. Since there are more than 12 hours of daylight in the Northern Hemisphere, that hemisphere receives more energy and warms up. The Southern Hemisphere suffers the opposite effect and cools off. There are four parallels of latitude that have been given special names in connection with the sun's two extreme positions. When the sun is at the summer solstice, it can be seen for 24 hours during the day within the cap bounded by the Arctic Circle, which lies 23½º from the North Pole (at 67½ºN latitude). This is the region of the midnight sun. At the same time, within the Antarctic Circle, 23½º from the South Pole (at 67½ºS latitude) there will be 24 hours of darkness during the day. In the winter, when the sun is at the other solstice, the circumstances in the caps reverse, and the Antarctic Circle defines the region of the midnight sun. The region on Earth, where on one or two days during the year, the sun can appear directly overhead, at the zenith, is bounded by tile so-called Tropics. The Tropic of Cancer is at 23½ºN and the Tropic of Capricorn is at 23½ºS. Their names were chosen by the early Greeks because the sun was entering the constellations of Cancer in the summer and Capricorn in the winter. Of course, that is no longer true today because of the precession of the equinoxesbut the names have stuck.
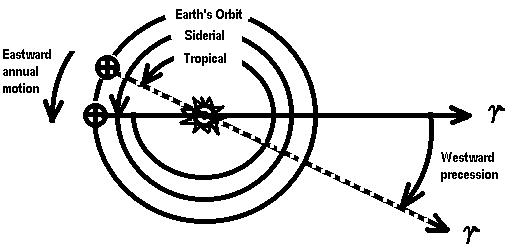 |
FIGURE 18 Comparison of the sidereal and the tropical years. Because the vernal equinox precesses westward, it does not take as long for the sun to appear to line up with the vernal equinox as it does with a star.
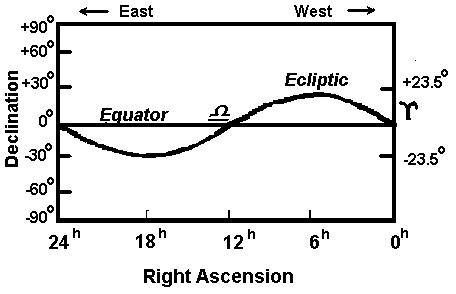 |
FIGURE 19 Plot of the ecliptic on the celestial sphere showing the locations of the equinoxes and the solstices.
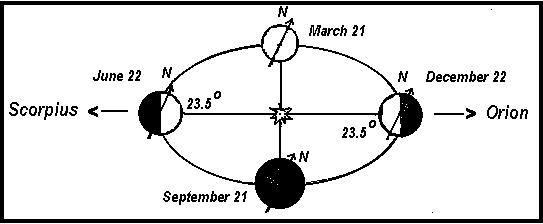 |
FIGURE 20 Causes of the seasons. During the summer in the Northern Hemisphere. The number of daylight hours exceeds the number of nighttime hours, and sunlight also strikes the surface of the Earth more directly than during the winter. Both of these effects contribute to supplying more solar energy to the Northern Hemisphere, making the summertime warmer than wintertime.
Type of Calender |
Average Length (days) |
| Early Roman: 365 days each year | 365.000000 |
| Julian: Every year divisible by 4 is a leap year. | 365.250000 |
| Gregorian: Century years not divisible by 400 are ordinary years. | 365.242500 |
| Modified Gregorian: The years 4000, 8000, 12,000 etc. are ordinary years. | 365.242250 |
| Tropical Year | 365.242199 |
TABLE 1
Comparison of various calendars.
Along with the increased length of the day, the rays of the sun strike the Earth's surface more directly in the Northern Hemisphere from the time of tile vernal to tile autumnal equinox, which results in more effective heating and adds to the seasonal changes. The opposite is true in the other half of the year. Both the variation in daylight hours and the changing angle at which sunlight strikes the Earth's surface result from the obliquity, of the ecliptic and are the detailed causes of the seasons.
The calendar was devised to count days and identify events according to the year. The early Roman calendar, from which ours is derived, contained 365 days per year and had no leap years. Because the number of days in a tropical year is not an exact number, the day of the beginning of spring continually fell later in the year. In 720 years, it would have moved from what is now March into September. Sosigines, an astronomer from Alexandria in Egypt, pointed out that the tropical year is about 365.25 days long. By adding a leap year of 366 days every fourth year, the average calendar year would become 365.25 days. Julius Caesar accepted Sosigines' observation and instituted the leap year in what we now call the Julian calendar. Of course, the tropical year is actually slightly shorter than 365.25 days. The time of the vernal equinox now moved more slowly through the calendar, but occurred progressively earlier in the year. In 1582 Pope Gregory, following the advice of the astronomer Clavius, introduced the Gregorian calendar. He deleted three leap years every 400 years by making century years not divisible by 400 into ordinary years. The average calendar year, over a 400-year span, becomes 365.2425 days, which is still slightly too long. As a point of interest, England and the American colonies did not adopt the Gregorian calendar until 1752. So the year 2000 will be the first century year that will be recognized as a leap year in Canada and the United States since the Julian calendar was abandoned. A modified Gregorian calendar has been proposed in which the years exactly divisible by 4000 will be ordinary years, giving an average length of 365.24225 days. Although it is over 2000 years before this modification will be required, it will result in a calendar in which the seasons will get out of step with the calendar by only 1 day every 19,600 years.
Here are references to recent books and WEB sites which discuss the history of time keeping and mathematical schemes for calculating latitude and longitude using a pocket calculator. You may use these as a supplement to the class material,. They are mostly for your enjoyment and entertainment.
BOOKS:
WEB SITES:
| daylight saving time | obliquity of the ecliptic |
| declination | parallel of declination |
| equinox | parallel of latitude |
| Foucault pendulum | precession |
| geographical latitude | prime meridian |
| geographical longitude | right ascension |
| Gregorian calendar | sidereal year |
| hour circle | solstice |
| Julian calendar | standard time |
| local apparent solar time | stellar aberration |
| local hour angle | tropical year |
| local mean solar time | universal time |
| local sidereal time | - |
1. What phenomenon does the Foucault pendulum demonstrate?
2. What are two methods observers on Earth can use to show that the Earth goes around the sun?
3. What causes the equatorial bulge of the Earth?
4. What causes the precession of the Earth's axis?
5. What are three motions of the Earth?
6. The North Star, Polaris, is within 1 degree of the North Celestial Pole. If Polaris appears about 40 degrees above your horizon, where are you on the Earth?
7. The following names refer to markings on a globe of the Earth. For each, list the corresponding name on the celestial sphere. a. Equator b. North Pole c. Latitude d. Longitude.
8. Latitude 0 degrees and declination 0 degrees occur on the terrestrial and celestial equators. These circles, in turn, are naturally determined by the terrestrial poles and the celestial poles. But longitude 0 degrees and 0 hours right ascension are arbitrarily defined and were selected for convenience. What defines longitude 0 degrees and 0 hours right ascension?
9. How many degrees does the celestial sphere appear to rotate in one hour? In four minutes?
10. The sun's diameter in the sky is about 0.5 degree. About how long does it take for the sun in its daily motion to appear to move its own diameter across the sky?
11. What is sidereal time?
12. Why has the keeping of accurate time traditionally been the task of astronomers?
13. What time is measured by a sundial?
14. Why is a solar day longer than a sidereal day?
15. Why don't we use local mean solar time in our everyday lives?
16. Why do standard time zones run generally north-south, not east-west?
17. The time zones for New York and California differ by 3 hours? When it is noon in New York, what time is it in California?
18. Why do different constellations appear at different seasons of the year?
19. What causes the seasons?
20. If you are north of the Arctic Circle in June (but not exactly at the North Pole), in what direction would you look to see the midnight sun?
21. How are the sidereal and tropical years defined?
22. What are the equinoxes and the solstices? When does the sun reach each of these points?
23. Why do we have leap years?