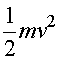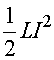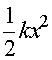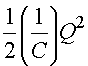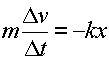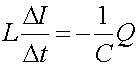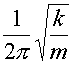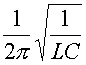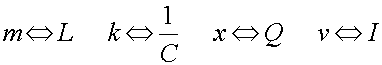LC circuits
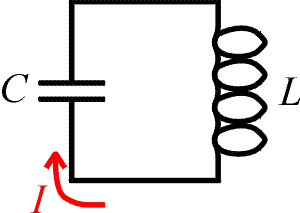 An LC circuit is a closed loop with just two
elements: a capacitor and an inductor. It has a resonance property
like mechanical systems such as a pendulum or a mass on a spring: there is a
special frequency that it likes to oscillate at, and therefore responds
strongly to. LC circuits can be used to tune in to a
specific frequency, for example in the station selector of a radio or television.
An LC circuit is a closed loop with just two
elements: a capacitor and an inductor. It has a resonance property
like mechanical systems such as a pendulum or a mass on a spring: there is a
special frequency that it likes to oscillate at, and therefore responds
strongly to. LC circuits can be used to tune in to a
specific frequency, for example in the station selector of a radio or television.
In an LC circuit, electric charge oscillates back and forth just like the position
of a mass on a spring oscillates. To demonstrate the analogy, we list several
corresponding equations for a mechanical spring and an LC circuit.
The parameters that determine the motion of a spring are the mass m,
spring constant k, the position x, and
the velocity v which is the rate of change of x.
The parameters that determine the behavior of an LC circuit are L,
C, Q and I
which is the rate of change of Q. Thus there is a one-to-one
correspondence since the equations of motion are identical given the substitutions:
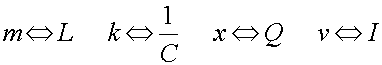
The characteristic frequency of an LC
circuit is the frequency at which large amplitudes are built up when a
driving force is applied at that frequency. A child on a swing will be
sensitive to a pushing force which comes regularly with the natural
frequency of the swing. A force that comes at a different frequency
will not build up a large amplitude as it will often be pushing against
the child's motion. The magic frequency is called
the resonant frequency.
Examples
AC circuits index
Lecture index
 An LC circuit is a closed loop with just two
elements: a capacitor and an inductor. It has a resonance property
like mechanical systems such as a pendulum or a mass on a spring: there is a
special frequency that it likes to oscillate at, and therefore responds
strongly to. LC circuits can be used to tune in to a
specific frequency, for example in the station selector of a radio or television.
An LC circuit is a closed loop with just two
elements: a capacitor and an inductor. It has a resonance property
like mechanical systems such as a pendulum or a mass on a spring: there is a
special frequency that it likes to oscillate at, and therefore responds
strongly to. LC circuits can be used to tune in to a
specific frequency, for example in the station selector of a radio or television.