Capacitance of two parallel plates The most common capacitor consists of two parallel plates. First, we derive the capacitance which depends on the area of the plates A and their separation d. According to Gauss's law, the electric field between two plates is:
Since the capacitance is defined by
Thus you get the most capacitance when the plates are large and close together. If a dielectric material is inserted between the plates, the microscopic dipole moments of the material will shield the charges on the plates and alter the relation. Materials have a permeability e which is sometimes given by the relative permeability k, e=ke0. The capacitance is then given by:
|







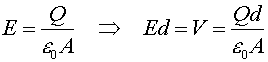
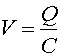 one can see that capacitance is:
one can see that capacitance is: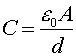
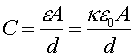
 All materials have a relative permeability, k, greater than unity, thus the capacitance
can be increased by inserting a dielectric. Sometimes, k is referred to as the dielectric constant of
the material. The electric field causes some fraction of
the dipoles in the material to orient themselves along
the E-field as opposed to the usual random orientation.
This, effectively, appears as if negative charge is lined
up against the positive plate, and positve charge against
the negative plate. In the figure to the right, the blue
material is the dielectric.
All materials have a relative permeability, k, greater than unity, thus the capacitance
can be increased by inserting a dielectric. Sometimes, k is referred to as the dielectric constant of
the material. The electric field causes some fraction of
the dipoles in the material to orient themselves along
the E-field as opposed to the usual random orientation.
This, effectively, appears as if negative charge is lined
up against the positive plate, and positve charge against
the negative plate. In the figure to the right, the blue
material is the dielectric.